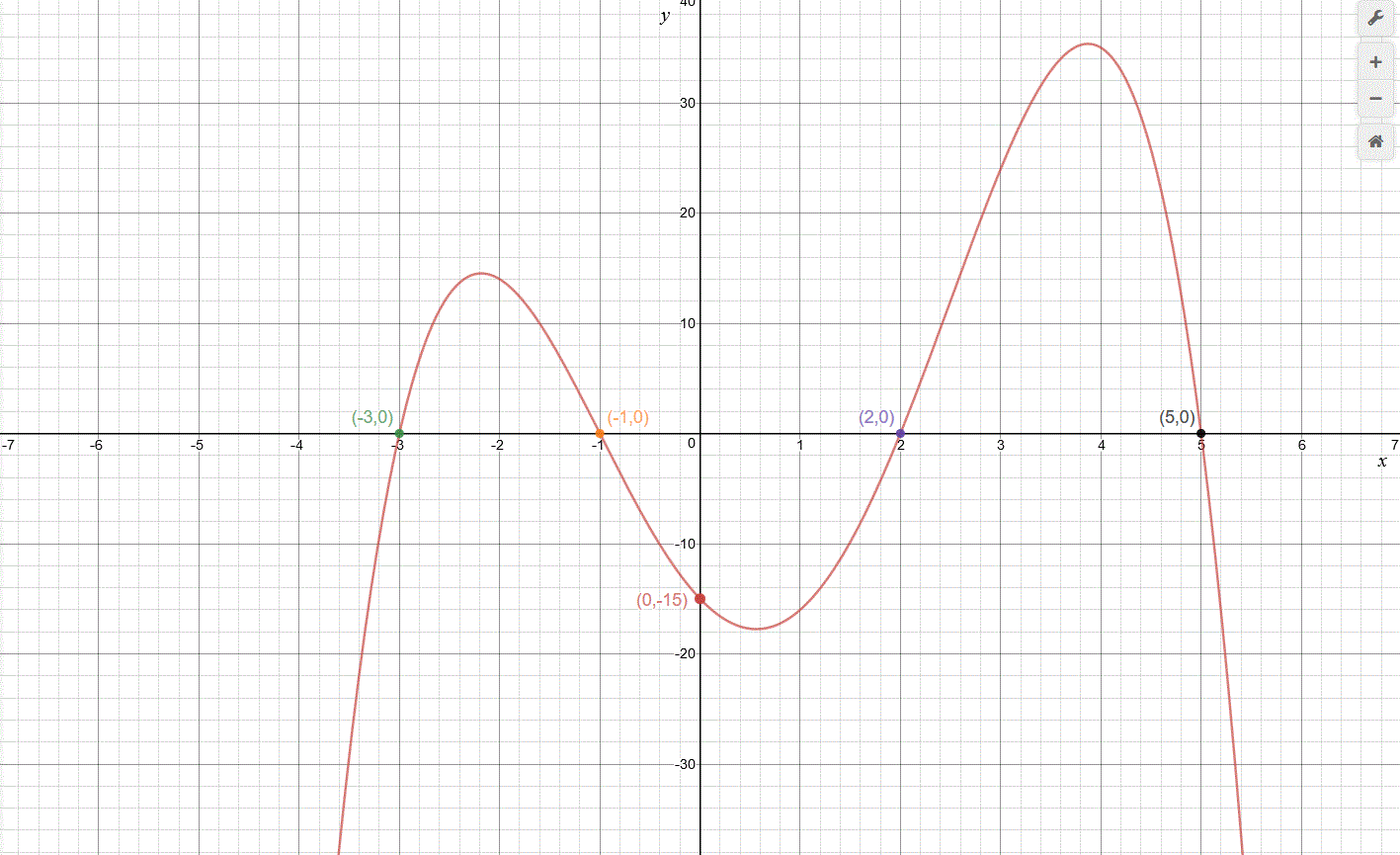
- degree: even
coefficient: negative 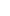
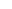 intercepts
intercepts
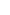 direction changes
direction changes

- The minimum degree is
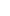
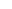 -intercepts are:
-intercepts are: 
Written as factors:

- Plug in the point
 and solve for
and solve for 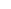





- The function for this graph is

or

Graphs of Polynomial Functions
Macy Maynard
